ISEE Mathematics Achievement Question 285: Answer and Explanation

Home > ISEE Test > ISEE Mathematics Achievement Practice Tests

Test Information

Question: 285

15.

In trapezoid LMNO, line LO has a length of 20. What is the area of the trapezoid?

  • A. 48
  • B. 64
  • C. 88
  • D. 112

Correct Answer: D

Explanation:

D Break the trapezoid into two triangles and a rectangle. Next, figure out the missing segment lengths. If MN = 8, then QP = 8. That means that 20 - 8 = 12, which is what LQ and PO must total. If each segment were the same, then LQ = 6 and PO = 6 since 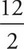= 6. Next, find out QM and PN. You may notice that these are 6-8-10 right triangles. Otherwise, use the Pythagorean Theorem to find the height of the triangles: 62 + b2 = 102. Solve for b: 36 + b2 = 100; subtract 36 from both sides to get b2 = 64. Then take the square root of both sides, and b = 8. To find the area of the triangles, plug in the base and height into the formula for the area of a triangle: A = bh = (6)(8) = 24. There are two triangles, so 24 + 24 = 48. Eliminate (A) since you haven't finished finding the area of the whole trapezoid yet. The rectangle QMNP is actually a square since all sides equal 8. To find the area of a square, use the formula: A = s2 = 82 = 64. Finally, add the areas: (2 triangles) + (1 square) = 48 + 64 = 112. The correct answer is (D).

Previous       Next